find the total kinetic energy ktot of the dumbbell.|10.4 Moment of Inertia and Rotational Kinetic Energy

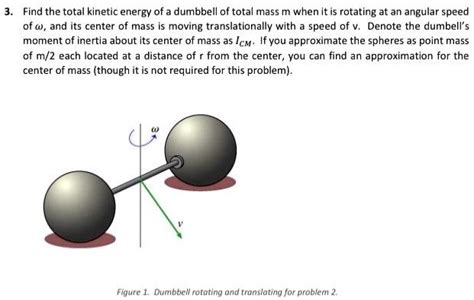
find the total kinetic energy ktot of the dumbbell.,You found that the total kinetic energy is the sum of the kinetic energy in the center of mass plus the kinetic energy of the center of mass. A similar decomposition exists for angular .Physics. Physics questions and answers. Due: 11 Kinetic Energy of a Dumbbell Part A Score: This problem illustrates the two contributions to the Find the total kinetic energy Ktot of the dumbbell Express your answer .Physics. Question. Find the total kinetic energy Kₜₒₜ of a dumbbell of mass m when it is rotating with angular speed ω and its center of mass is moving translationally with speed .VIDEO ANSWER: So in this question, we have to fund a total kinetic energy of a dumbbell. And dumbbell is like this. So it is given the center of mass is moving with .

This free textbook is an OpenStax resource written to increase student access to high-quality, peer-reviewed learning materials.Learn how to calculate the rotational kinetic energy and the moment of inertia of a rigid body with OpenStax, a free and high-quality textbook provider.The total kinetic energy of the dumbbell can be calculated by adding the translational kinetic energy and the rotational kinetic energy. Step 2/5. The translational kinetic .find the total kinetic energy ktot of the dumbbell. 10.4 Moment of Inertia and Rotational Kinetic Energy Key Concepts. previously, we have found that a single point (CM) can be used to describe the bulk kinematic properties of an object. now we will discuss one of the common .

The key effect of this constraint is that both masses rotate about the axle with the same angular frequency ω. If the particles are respectively distances d 1 and d 2 from the axle, then their speeds are .

You are to find the total kinetic energy Ktotal of a dumbbell of mass m when it is rotating with angular speed ω and its center of mass is moving translationally with speed v. (Figure 1) Denote the dumbbell's . but this fact will not be necessary for this problem. Part A Find the total kinetic energy Ktot of the dumbbell. Express your answer .

Find the total kinetic energy of a dumbbell of total mass m when it is rotating at an angular speed of w, and its center of mass is moving translationally with a speed of v. Denote the dumbell's moment of inertia about its center of mass as Icm. If you approximate the spheres as point mass of m/2 each located at a distance of r from the center .Question: This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy Ktotal of a dumbbell of mass m when it is rotating with angular speed w and its center of mass is moving translationally with speed v.

View Available Hint(s) Ktot Immet This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy Ktotal of a dumbbell of mass m when it is rotating with angular spoed w and its center of mass is moving .This problem illustrates the two contributions to the kineticenergy of an extended object: rotational kinetic energy andtranslational kinetic energy. You are to find the total kineticenergy of a dumbbell of mass when it is rotating with angular speed and its center of mass ismoving translationally with speed .You are to find Find the total kinetic energy Ktot of the dumbbell. Express your answer in terms of m, v, Icm, and w tional kinetic the total kinetic energy Ktotal of a dumbbell of mass m Hint when it is rotating with angular speed and its center of mass is moving translationally with speed v. (Figure 1 Denote the dumbbell's moment of inertia .

This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy K total of a dumbbell of mass m when it is rotating with angular speed omega and its center of mass is moving translationally with speed upsilon.10.4 Moment of Inertia and Rotational Kinetic Energy The kinetic energy and angular momentum of the dumbbell may be split into two parts, one having to do with the motion of the center of mass of the dumbbell, the other having to do with the motion of the dumbbell relative to its center of mass. To do this we first split the position vectors into two parts. The centre of mass is at.This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy Ktotal of a dumbbell of mass mm ω and its center of mass is moving translationally with speed vv (Figure 1)Denote the dumbbell's moment of inertia about .You found that the total kinetic energy is the sum of the kinetic energy in the center of mass plus the kinetic energy of the center of mass. A similar decomposition exists for angular and linear momentum. . not just rigid bodies like a dumbbell. It is important to understand the applicability of the formula Ktot=Kr+Kt. Which of the following .find the total kinetic energy ktot of the dumbbell.You are to find the total kinetic energy of a dumbbell of mass when it is rotating with angular speed and its center of mass is moving translationally with speed. Denote the dumbbell's moment of inertia about its . Ktot = 0 v 2 + 0ω 2 Ktot = Kr +Kt The velocity vector v⃗must be perpendicular to the axis of rotation.

This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy Ktotal of a dumbbell of mass m when it is rotating with.

This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy K t o t a l of a dumbbell of mass m when it is rotating with angular speed ? and its center of mass is moving translationally with speed v.Transcribed Image Text: 10 Kinetic Energy of a Dumbbell This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy Krotal of a dumbbell of mass m when it is rotating with angular speed w and its center translationally with .Find step-by-step Physics solutions and your answer to the following textbook question: Find the total kinetic energy Kₜₒₜ of a dumbbell of mass m when it is rotating with angular speed ω and its center of mass is moving translationally with speed v. Denote the dumbbell's moment of inertia about its center of mass by I꜀ₘ. **Note:** If you .

Question: This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy Ktotal of a dumbbell of mass m when it is rotating with angular speed ω and its center of mass is moving translationally with speed v.However, because kinetic energy is given by K = 1 2 m v 2 K = 1 2 m v 2, and velocity is a quantity that is different for every point on a rotating body about an axis, it makes sense to find a way to write kinetic energy in terms of the variable ω ω, which is the same for all points on a rigid rotating body. For a single particle rotating .

find the total kinetic energy ktot of the dumbbell.|10.4 Moment of Inertia and Rotational Kinetic Energy

find the total kinetic energy ktot of the dumbbell.|10.4 Moment of Inertia and Rotational Kinetic Energy .

Share:

×

Share

Copy Link

Email

Facebook

Twitter

LinkedIn

WhatsApp

Download: Full Size (80225 MB)

Photo By: find the total kinetic energy ktot of the dumbbell.|10.4 Moment of Inertia and Rotational Kinetic Energy

VIRIN: 44523-50786-27744